
If some function is applied to both sides of an equation, the resulting equation has the solutions of the initial equation among its solutions, but may have further solutions called extraneous solutions. For a system: adding to both sides of an equation the corresponding side of another equation, multiplied by the same quantity.For example, expanding a product or factoring a sum. Applying an identity to transform one side of the equation.Multiplying or dividing both sides of an equation by a non-zero quantity. The field of mathematics covers a wide range of applications, from simple arithmetic operations to complex equations.This shows that every equation is equivalent to an equation in which the right-hand side is zero. Adding or subtracting the same quantity to both sides of an equation.The following operations transform an equation or a system of equations into an equivalent one – provided that the operations are meaningful for the expressions they are applied to: Two equations or two systems of equations are equivalent, if they have the same set of solutions. In this example, restricting θ to be between 0 and 45 degrees would restrict the solution to only one number.

into my calculator, FOIL worksheet multiple choice, simple maths formulas. Since the sine function is a periodic function, there are infinitely many solutions if there are no restrictions on θ. Solving equations worksheets, Prentice Hall Mathematics Geometry answers. For example, the equationĪ x 2 + B x + C − y = 0 The sides of a polynomial equation contain one or more terms. The most common type of equation is a polynomial equation (commonly called also an algebraic equation) in which the two sides are polynomials. Assuming this does not reduce the generality, as this can be realized by subtracting the right-hand side from both sides. Very often the right-hand side of an equation is assumed to be zero. The expressions on the two sides of the equals sign are called the "left-hand side" and "right-hand side" of the equation.
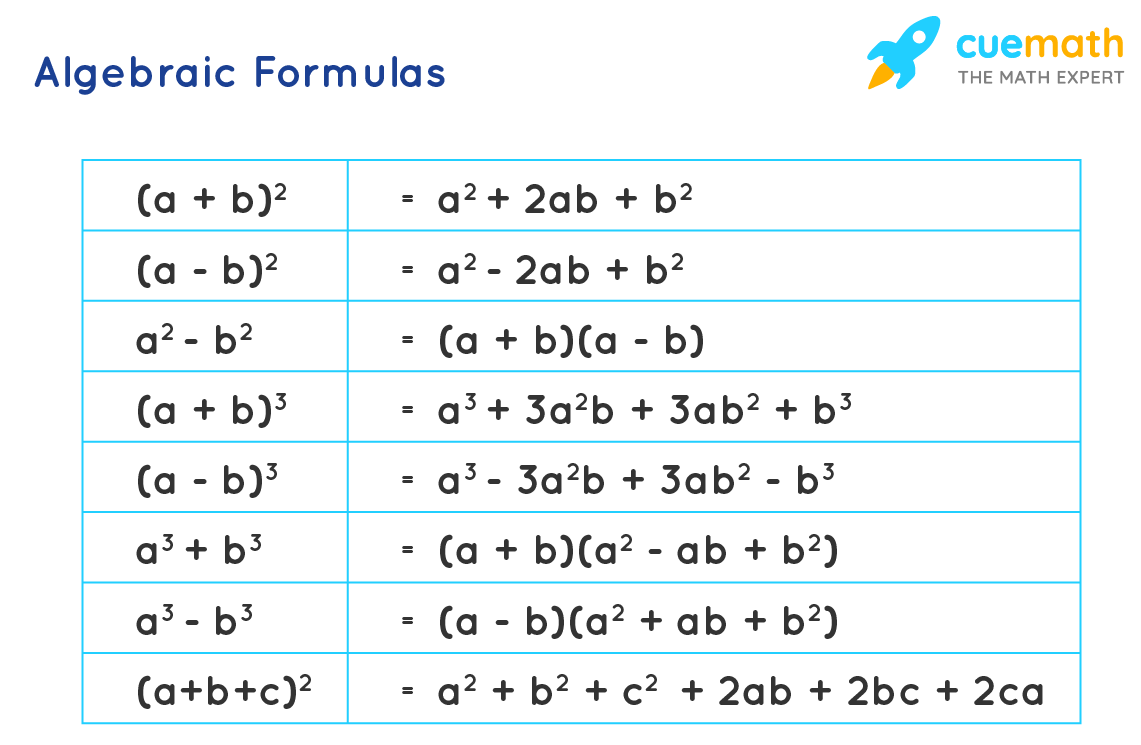
Īn equation is written as two expressions, connected by an equals sign ("="). A conditional equation is only true for particular values of the variables. An identity is true for all values of the variables. There are two kinds of equations: identities and conditional equations. The variables for which the equation has to be solved are also called unknowns, and the values of the unknowns that satisfy the equality are called solutions of the equation. Solving an equation containing variables consists of determining which values of the variables make the equality true.

The word equation and its cognates in other languages may have subtly different meanings for example, in French an équation is defined as containing one or more variables, while in English, any well-formed formula consisting of two expressions related with an equals sign is an equation. In mathematics, an equation is a formula that expresses the equality of two expressions, by connecting them with the equals sign =. From The Whetstone of Witte by Robert Recorde of Wales (1557). The first use of an equals sign, equivalent to 14 x + 15 = 71 in modern notation.


 0 kommentar(er)
0 kommentar(er)
